How far away is the
horizon?
We know that the ancient Greeks were well aware of the curvature of the
earth even several centuries B.C. Ptolemy made a map of the known world(150AD).
However, from our discussion about
the flat earth model in the Zhou bi suan, even into the 17th century
A.D. there were competing models of terrestrial geometry in
China. What advantage did the Greeks have to arrive at the spherical
earth model thousands of years before the Chinese? Perhaps we can
understand this because of the maritime nature of Greek life. Being a
nation so closely dependent on the sea they soon realized that the
earth could not be flat and that it must have the shape of a sphere.
This is a fact readily attested to by anyone who pays attention to the
appearance of objects at sea when the horizon is visible. The horizon
is a line clearly demarcating the separation of the sky and the sea.
Consider an observer standing on a high pier watching sailboats, as in
Figure 1. Three identical boats are at sea, A, B, C. The observer sees
only the mast of boat A, all of boat B, and the mast of boat C is cut
by the horizon. These features can only be explained by assuming a
curvature to the water's surface so that only the mast of boat A is
visible because the hull of this boat is obscured by the curvature of
the earth.
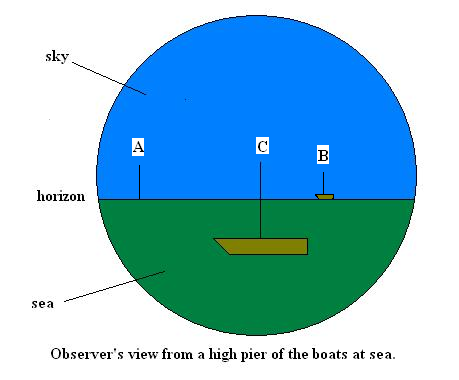
Figure 1
An interesting geometrical question now arises as to how far away is
the horizon? This can be answered by using the observations on boat C
as shown in figure 2.
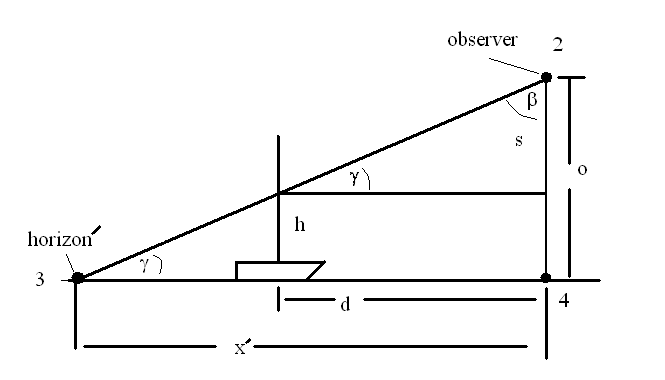
Figure 2
The observer on the pier is at point 2, a distance o above the water.
Point 4 is directly below point 2 and points 3 and 4 are assumed to be on the water
surface. The boat C is a distance d away from the pier. By sighting the
horizon' at point 3 the line of sight cuts the mast at a distance h
above the water. If the observer knows the distance d, the heights o
and h then since there are two similar right triangles :
x'/o = d/s and this yields x' = d*o/(o - h),
where s = (o - h). The trigonometry for Figure 2 also shows that
tan(gamma) = s/d. One sees that as x' grows larger that the angle beta
approaches 90 degrees. If the horizon' were really infinitely far away
then beta = 90 degrees and we could never see the mast being cut by the
horizon if h < o. Flat earth proponents would then conclude that
after sailing the distance x' they would come to the edge of the earth.
The question now is how far away is the horizon if we use the round
earth model? In practice this means for an object floating on the
surface of the water, how far away is it so that we can just barely see
it? For this question we study Figure 3.
Here the radius of the earth is R. The triangle 234 is again shown.
Notice that the line 34
is perpendicular to 24
. What we called the distance x' in figure 2 is the length of line 34. The horizon' is then at
point 3 where the line of sight 21 intercepts line 34. The true horizon is at
point 1, the tangent point for the line of sight from point 2. Again we
have two similar right triangles, 234 and 1C2. The distance along the
surface of the curved earth is
x = gamma*R, where angle gamma is measured in radians. From the
trigonometry we see
cos(gamma) = R/(R+o).
So for R = 6378 km = 6.378 x 106 m, and for an
observer standing at the water's edge of height o = 2m,
gamma = 7.92 x 10-4 radians and x = 7.92 x 10-4 * 6378 km = 5
km. This is a surprisingly small distance. The horizon is not far away
for the average person strolling along the beach. It also indicates why
the crow's nest on a ship is needed to see greater distances. At a
height of 10m above the water the horizon is 11.3 km away. Since any
practical measurement will require o << R we can get a very good
approximation to the angle gamma by expanding the cosine and the ratio
R/(R+o):
gamma = sqrt(2*o/R) for o << R.

Figure 3: Round earth model for the distance to the horizon.
Now we can understand the advantage a sea faring people have over a
land locked people in determining the geometry of the earth. In the
middle of the continent we can see very much farther since there is a
great variation of topography when mountains are visible. A person in a
valley would have difficulty in assessing the earth's shape if his
range were only tens of kilometers. We can see the curvature on
land but it requires a great deal more knowledge. For example, Pikes
Peak west of Colorado Springs is at an elevation of 4300m above sea
level. Driving west on I70 in Colorado eventually brings Pikes Peak
into view, but the top of Pikes Peak is seen first before the whole
mountain, just as is the case in Figure 1 where only the top of the
mast is seen for boat A. The average Colorado elevation on I70 between
the Kansas border and Colorado Springs is about 1500m. The difference
in elevation between Pikes Peak and the average elevation on I70 is
about 2800 m. In order for Pikes Peak to be visible we can us our
equations above. In this case we have the observer on the summit of
Pikes Peak looking for cars on I70, hence o = 2800 m.
gamma = sqrt(2*2800m/6.378 x 106 m) = 0.0296 radians.
x = 0.0296*6378 km = 190 km.
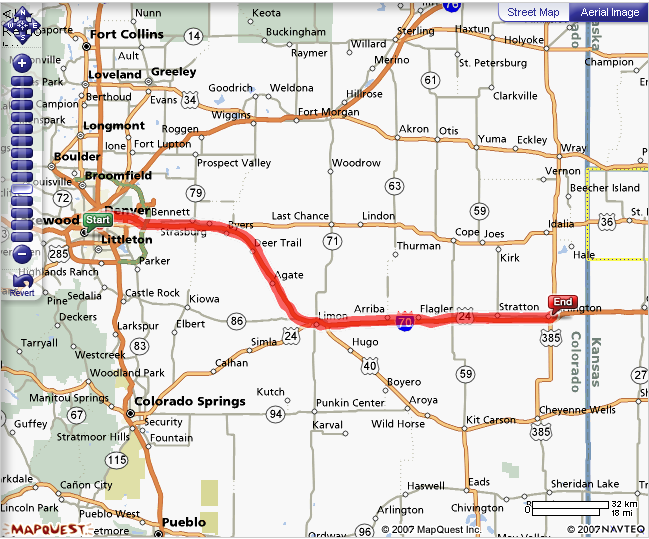
So from the map below the driver on I70 should start seeing the summit
of Pikes Peak about 190 km east of Colorado Springs, or about at
Flagler, CO.
We have neglected atmospheric refraction in this discussion. It should be a negligible effect for small observer heights. Can you see how the derivation here could be turned around to determine the radius of the earth?